Capacitance exists from every surface in a cable to all other surfaces in and around cable. An understanding of the relative amount of capacitance to be expected in a cable is helpful in specifying cable and designing terminal equipment for the cable to be used. Although capacitance in a cable may have negligible effect on DC or 60 cycle AC cable circuits used for power or control, it does affect higher frequency AC voltages.
Charge on Conductive Surfaces
Elementary electron theory states that the electron is the basic unit of negative electric charge; that a large charge is simply a large collection of electrons. The more electrons that are concentrated on a surface, the larger the electric charge on that surface. Positively charged surfaces may be regarded as having a deficiency of electrons. Charge is measured in coulombs. One coulomb consists of many trillions of electrons. Since they repel each other, a collection of electrons will distribute over a surface.
Current
We can force an exchange of charge (electrons) between two conductive surfaces if we connect any source of voltage, such as a battery, between them. Since electrons in motion constitute an electric current, current flows off one surface through the battery onto the other, until the repulsion of charges being forced onto the surfaces equals the forcing voltage. When the battery is removed, a voltage equal to that of the battery exists between the surfaces due to the stored charge. If the voltage source is AC, the charge exchange occurs each half cycle. The voltage source thus must handle this charging current in addition to any other current which may pass through circuitry connected to the two surfaces.
Capacitance
This behavior of charge on surfaces is termed capacitance. Capacitance may be defined as the ratio of voltage between two surfaces, divided by their difference in charge; and is measured in units called farads. Capacitors, or condensers, are sets of surfaces deliberately arranged to control the capacitance between them. Shielded wires and cables also have capacitance between the conductors and shields which should be considered in their design and application. Commonly used values of capacitance are microfarads (mfd) or (10-6 fd) and picofarads (pf) or (10-12 fd).
Top of Page
Dielectrics
The spacing or insulating material between two surfaces of a capacitor is called a dielectric. It may be vacuum, air, or one of many insulating materials. With the exception of gases, all insulating materials increase the capacitance between the surfaces. The term dielectric constant is used to show how large this effect is for various materials. If the space between two surfaces is filled with a material having a dielectric constant of 2, then the capacitance between the surfaces will be 2 times greater than it would be for an air or vacuum dielectric.
Wire Insulation
Not all dielectrics are suitable for use on wire and cable. The more commonly used insulation's are listed in Table A. Most wire insulation choices are based on a compromise among cost, electrical performance, and the physical and chemical properties required for the application. With the exception of Teflon® and some polyolefins, most wire insulation's exhibit appreciable increases in their dielectric constant and insulation leakage with increasing temperature or frequency. This may make them undesirable for use where the capacitance, characteristic impedance or the leakage must be constant, such as in coaxial cable or instrumentation cables.
Geometry
The shape, diameter and spacing of the conductors and shields determine the capacity between them. Coaxial cables are a special application version of a single shielded conductor and may be treated in the same way.
Top of Page
Coaxial Wires
The capacitance per foot between a single insulated wire and a shield around it is:
Where e is the dielectric constant of the insulation between wire and shield; and D/d is the diameter ratio of shield ID to conductor OD. The only way in which the capacitance can be selected is by choice of insulation having a low dielectric constant, or by choosing a suitable value of D/d.
Top of Page
Shielded Pairs
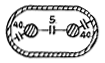 (Ref. to Mil-C-17.)
(Ref. to Mil-C-17.)
Shielded pairs have three capacitance's involved which combine to produce the effective wire to wire capacitance. Fig. 2 illustrates these capacities. Since the wire-to-shield capacitance's of each conductor are essentially in series, the effect of the two 40, mmfd capacitance's is to produce an apparent 20 mmfd between the two wires in addition to the 5. mmfd which will exist whether the shield is present or not. Thus the effective capacitance is 20. + 5. = 25. mmfd./ft from wire to wire.
Top of Page
Multi-Conductor Cable Bundles
The capacitance from a wire to all else in a large cable bundle of identical wires may vary widely depending on insulation and geometry. In general, however, it will have values ranging from 40. to 65. mmfd/ft for PVC insulation (where C=4.), and will vary from this for other materials. Conductors in the outer layer of a cable bundle which is overall-shielded will tend to have a higher capacitance than those closer to the bundle center. Typical variation for PVC' insulated wires will be a 15-20% rise in capacitance for conductors in the layer closest to the overall shield.
Inductance
When current flows in a wire it creates a magnetic field about the wire, which generates voltages along the same wire as the current changes. These opposing voltages act to limit the rate at which the current can change. This effect is termed inductance and is measured in units called henrys. The self inductance of a round straight copper wire is on the order of .4 micro-henry/ft, and is relatively unaffected by diameter or length of wire. The self inductance of twisted pairs of wires is on the order of .08 micro-henry/ft; while the mutual inductance of a coaxial construction is .14 Lg10(D/d) micro-henrys/ft.
Mechanical Delay
The electronic systems designer should consider possible problems which may arise due to cable delay. For instance, in a multi-conductor cable of coax, the coax in the center of the bundle will be shorter than those in the outer layers by 4% to 6%, which mechanically can introduce delay in the signals traveling the long path.
Top of Page
Dielectric Delay
Although radio waves travel at the speed of light in free space or in air, this speed is much less when the wave is guided through coax or other shielded cables, where the electric field is contained in an insulator other than air.
Suppose that a radio-frequency sine-wave signal generator is connected to both an antenna and a 1000 foot length of coaxial cable, so that its signals will be launched simultaneously into both, and we go to the far end of the cable to see which arrives first.
When the generator is keyed on, the signal from the antenna arrives first, traveling at the speed of light in air, taking about one microsecond to make the trip.
Shortly thereafter, the same identical signal will arrive at the end of the coax cable, having taken longer to travel the same distance. It did not travel as fast, so its arrival at the end of the cable was "delayed" compared to the arrival of signal from the antenna.
The velocity of a wave in a coax is usually expressed as a percentage of the velocity of light. For instance, a polyethylene insulation gives a "propagation velocity" of 65.9% of light velocity. This is sometimes expressed as a velocity factor of .659.
| TABLE A |
| Insulation |
(e) |
Prop. Velocity: v c |
Transit time micro-sec/1000 ft. |
| Air |
--**** |
Nom |
1.06**** |
| Polyethylene |
2.26 |
65.9% |
1.54 |
| Teflon (TFE & EEP) |
2.0 |
70.0% |
1.45 |
| Polypropylene |
2.0 |
70.0% |
1.45 |
| Foam Polyethylene |
1.55 |
80.0% |
1.27 |
| Polyvinylchloride |
4.0*** |
50.0% |
2.03 |
| Nylon (#610) |
3.0** |
57.8% |
1.76 |
| Neoprene |
5.0*** |
70.0% |
1.06**** |
| Rubber (Buna S) |
2.9*** |
58.7% |
1.73 |
| Rubber (Butyl) |
2.35*** |
65.3% |
1.56 |
| Silicone |
3.16*** |
56.3% |
1.80 |
microsecond is one millionth of a second
**Susceptible to changes due to humidity. Absorbs moisture
***Dielectric constant varies widely with frequency Many different values of dielectric constant may be obtained since the materials are a blend of filler and plasticizers with the base material, all of which have differing values of e
****Varies depending on the method used to support the center conductor of the cable. Inductance of shield and conductor limit upper value to about 96%
|
Characteristic Impedance
A transmission line such as a coaxial cable or shielded pair can be considered as a wave-guiding device in the broad sense. The relative amplitudes of the electric and magnetic fields due to a signal within the cable are determined by the capacitance and inductance per unit length of cable (assuming no reflections from the load.)
The characteristic impedance (Z) is the ratio of the two fields, or 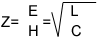 where C and L are in farads and henrys.
where C and L are in farads and henrys.
Another equation for Z is:  where C is in pf/ft and V.P. is the propagation velocity expressed in percent.
where C is in pf/ft and V.P. is the propagation velocity expressed in percent.
The resistance of the conductors and shield attenuates the signal in a transmission line, but at radio frequencies has little effect in determining the characteristic impedance.
Top of Page
Reference Books
Radio Engineers Handbook; Terman; McGraw-Hill
Pulse, Digital, & Switching Waveforms; Millman & Taub; McGraw-Hill
Reference Data For Radio Engineers; I. T. & T. Corp.; Stratford Press
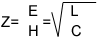 where C and L are in farads and henrys.
where C and L are in farads and henrys.

